Geometry Problems
For information, comment on the problems or their solution, please contact the artist
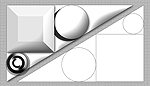
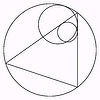
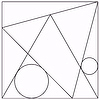
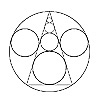
1 - From a 1803 Sangaku found in Gumma Prefecture. The base of an isosceles triangle sits on a diameter of the large circle. This diameter also bisects the circle on the left, which is inscribed so that it just touches the inside of the container circle and one vertex of the triangle. The top circle is inscribed so that it touches the outsides of both the left circle and the triangle, as well as the inside of the container circle. A line segment connects the center of the top circle and the intersection point between the left circle and the triangle. Show that this line segment is perpendicular to the drawn diameter of the container circle. (Tonyv Rothman) |
|
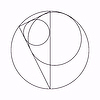
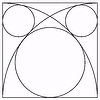
| 2 - 1788 Sangaku, Tokyo Prefecture. Also called the Pappus chain. It asks for the radius of the nth largest inner circle in terms of r, the radius of the container circle. Note that the two large vertical circles are identical, each with radius r/2. The original solution to this problem deploys the Japanese equivalent of the Descartes circle theorem. This remarkable theorem states that if one inscribes a chain of circles in the arbelos, the first circle in the chain being tangent to all three semicircles, and the subsequent circles in the chain being tangent to the preceding circle and to two of the semicircles, then the height of the center of the nth circle above the horizontal line segment AB is n times the diameter of that circle (Harold P. Boas) |
|
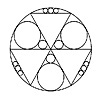
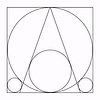
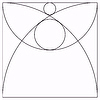
3 - The figure is symmetric. AD, BE and CF are the diameters of the circle O, and AOB = AOF = 60° . O1(a) and O4(b) touch each other externally, touch BE and CF, and O1 touches O internally. O7(c ) touches O8(d ) and O9(d ) externally. O7 , O8 and O9 touch AF and touch the circle O internally. Find d in terms of b . (Hiroshi Okumura) |
|
|
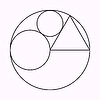
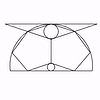
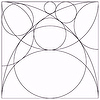
4 - If CB'A is an isosceles triangle with CB' = CA, and B is a point lying on the line B'A, then one of the circles touching the circumcircle of BCB' internally and also touching AB and AC is twice the size of the incircle of ABC. (Hiroshi Okumura) |
|
5 - 1874 tablet from Gumma Prefecture. A large circle lies within a square. Four smaller circles, each with a different radius, touch the large circle as well as the adjacent sides of the square. What is the relation between the radii of the four small circles and the length of the side of the square? The problem can be solved by applying the Casey theorem, which describes the relation between four circles that are tangent to a fifth circle or to a straight line. (Tony Rothman) |
|
|
6 - If CB'A is an isosceles triangle with CB' = CA, and B is a point lying on the line B'A, then one of the circles touching the circumcircle of BCB' internally and also touching AB and AC is twice the size of the incircle of ABC. (H. Okumura, M. Watanabe) |
|
7 - In a square PQRS, there are two circles touching SP and the incircle of the square, where one of which touches PQ and the other touches RS. Let A be the point of tangency of QR and the incircle and let the tangents of the two small circles through A intersect the segment SP at B and C. Given the inradius of the square, find the inradius of the circle in the triangle ABC. The answer is that the medium circle is also half the size of the largest circle. (Hiroshi Okumura) |
|
8 - Two congruent regular pentagons with a side in common are inscribed in a large circle. A circle of radius r touches the large circle and the sides DE and EF of the pentagons, and the incircle of the triangle ABH has radius t. Show that r = 2t. (John Rigby) |
|
9 -Two equilateral triangles are inscribed into a square. Their side lines cut the square into a quadrilateral and a few triangles. Find a relationship between the radii of the two incircles. (H. Fukagawa, D. Pedoe, Jill Vincent & Claire Vincent) |
|
10 - Find a relationship between the radius of the circle and the side of the square. The relative radii of the circles shown can be solved by an application of the Pythagorean theorem if 10, 11, 12 are combined into a single configuration. (Alexander Bogomolny) |
|
11 - Find the radius of (K) tangent to (A), (B) and AB.(Alexander Bogomolny) |
|
12 - Aichi Prefecture, 1877. ABCD is a square of side a, and M is the centre of a circle with diameter AB; CA and DB are arcs of circles of radius a and centres D and C respectively. Find the radius, r1, of the circle, centre O, inscribed in the area common to these circles. Find also the radius, r2, of the circle, centre O', which touches AB and touches CA and DB externally as shown. (Jill Vincent & Claire Vincent) |
|
|
13 - This configuration (10, 11, 12) was noted by L. Bankoff and C. W. Trigg after a 1998 Scientific American article, featuring numerous sightings of the famous 3:4:5 triangle. (Alexander Bogomolny) |
|
14 - Let T be the intersection of AI extended with (A). Then AT = 24 and, since AI = 20, IT = 4 = IQ. The circle (I) of radius 4 is tangent to (A), (B), (F), and (E). If we extend AT further and let R on AT be on the vertical tangent SR to (I), then, ΔIRS is similar to AEI and, hence, is also 3:4:5. Since IS = IQ = 4, IR = 20/3, SR = 16/3, RT = 8/3. If U is the intersection of SR and CD, then RU = FI - SR = 8 - 16/3 = 8/3 = RT. (Alexander Bogomolny) |
|
15 - Assuming htat the side of the square is 24 so that DF = FC = 12. A circle with center X will be denoted (X). AH is tangent to (F) in G. Thus also FG = 12. The radius FG extends to meet BC in J and is perpendicular to AH. HC = HG as tangents to (F) from H. AG = AD = 24. FH bisects CFG and FA bisects its supplementary angle DFG. From here, AFH = 90º and FG is the altitude to the hypotenuse of right ΔAFH. We, therefore, have FG(x2) = AG·GH, or 12 (x2) = 24·GH. Wherefrom GH = 6 and so HC = 6. Then HB = 18, AH = 30, AK = 15, KG = 9, EK = 9, FK = 15, QK = 3, DJ = 16, FI = 8, EI = 16, AI = 20 (by the Pythagorean theorem), HJ = 10 (ΔFGK is similar to ΔJGH), BJ = 8, GJ = 8, FJ = 20, CJ = 16, IQ = 4. Triangles FCJ, HGJ, FGK, AKE, AEI, DFI, and ABH are all 3:4:5 triangles. (Alexander Bogomolny) |
|
16 - Let T be the intersection of AI extended with (A). Then AT = 24 and, since AI = 20, IT = 4 = IQ. Which says that the circle (I) of radius 4 is tangent to (A), (B), (F), and (E). If we extend AT further and let R on AT be on the vertical tangent SR to (I), then, for one, ΔIRS is similar to AEI and, hence, is also 3:4:5. Since IS = IQ = 4, IR = 20/3, SR = 16/3, RT = 8/3. If U is the intersection of SR and CD, then RU = FI - SR = 8 - 16/3 = 8/3 = RT. In addition, if V is on BC and VC = RU, then VH = 10/3, RV = 8 and, by the Pythagorean theorem, HR = 26/3, so that again RX = 8/3. It follows that the circle (R) with radius 8/3 is tangent to (A), (I), (H) and CD. (Alexander Bogomolny) |
|
|
17 - Three small congruent circles, two of which lie in the curvilinear triangles made by the two external tangents and the medium circle. The other lies in one of the curvilinear triangles made by the two larger circles and one of the external tangents. The ratio of the size of the two larger circles is 2:1. If there are two externally touching circles with different radii with external common tangents and if there are 4n congruent small circles, n of which lie in the curvilinear triangle made by the two external tangents and the medium circle, and 3n of which lie in one of the curvilinear triangles made by the two larger circles and one of the external tangents, then the ratio of the size of the two larger circles is 4:1 for any natural number n - in this figure n = 1, 2. (Hiroshi Okumura) |
|
18 - A triangle ABC is inscribed in a circle O (R) . In ABC , circles O2 (r), O4 (r1), O5 (r2), etc... are tangent one after another, as shown. The circles O1 (t1) , O3 (t3) touch the circle O(R) and touch chords AC and BC . Find r1 , r2, r3, .... in terms of t1, t2 and r. (Hiroshi Okumura) |
|
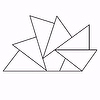
19 - Sangaku from the Miyagai prefecture, circa 1912. 6 congruent right triangles fan out along the sides of a regular pentagon of side a. Find the length of the hypotenuse t of these triangles in terms of a.(Jill Vincent & Claire Vincent) |
|
|
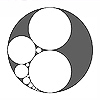
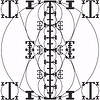
20 - Fractal image based on the fact that the ratio of the size of the two inscribed circles in the problem is 2:1. This provides an example of recursive computer programming. (Hiroshi Okumura) |
|
|